Rational numbers

  1. The numerical expression 3/7 + (-7)/8 = 25/56 shows that rational numbers are closed under addition.
  2. To prove that root 2 is an irrational number a teacher begins by assuming that it is a rational number and then proceeds to show how the assumption is not possible it is an example of contradiction
  3. Let a,b,c be 3 rational numbers , where a=3/5, b= 2/3 and C= -5/6 . The truth is a+(b+c)=(a+b)+c
  4. To prove that there is no rational number whose square is 2. This type of proof is 
    1. proof by contradiction
  1. According to Piaget, when the child is at the formal operational stage it is appropriate to introduce ratio and proportions.

Geometry:

  1. As per NCF,2005 Mathematics curriculum is ambitious, coherent and important to teachers. Here ambition refers to seek higher aims of teaching mathematics in school.
    1. In order to be coherent, the teacher has to teach a variety of mathematics like arithmetic, algebra, geometry and data handling.
  2. Geoboard is an effective tool to teach geometrical shapes and their properties.
  3. A geometric representation showing the relationship between a whole and its part is a pie chart.

NCERT:

  1. In NCERT exemplar book for class 8 at the end of a unit – 5, ‘understanding quadrilaterals and practical geometry’ lots of activities like constructing tessellation, constructing, tangrams, etc are given. One of the objectives of such a task is to help –
    1. all learners with different learning styles and to enhance spatial orientation.
  2. Two complementary angles are in the ratio 2:3. Find these angles. The above problem from NCERT textbook of class 7 refers to
    1. higher order thinking as it demands the interpretation of given information, its analysis and use in getting the desired information.

Theorem:

  1. ‘Representation’ in mathematics does not refer to
    1. expressing an important geometrical result as a theorem
      1. But it includes
        1. expressing the number sequence through geometrical patterns
        2. expressing the relation between 2 variables as an equation
        3. expressing the given data through graphs.
  2. Writing proofs in geometry implies – arguments or justification of statements.
    1. axioms are propositions which are assumed
    2. Axioms, when proved, become theorems
  3. Consider the following statement
    1. “If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram”. This statement is an theorem.
  1. In geometry class of 6-grade students, the teacher explained the construction of angles measuring 30 degrees, 60 and 90 degrees with the help of demonstration of construction and bisector of an angle. Then she asked the student to construct an angle of 15 degrees and an angle of 45 degrees. The task at this point reflects the teacher’s intention to assess the students understanding and ability to combine 2 skills learned to accomplish the given task.
  2. With the help of GeoGebra software, students can learn all concepts of geometry through an exploratory approach.
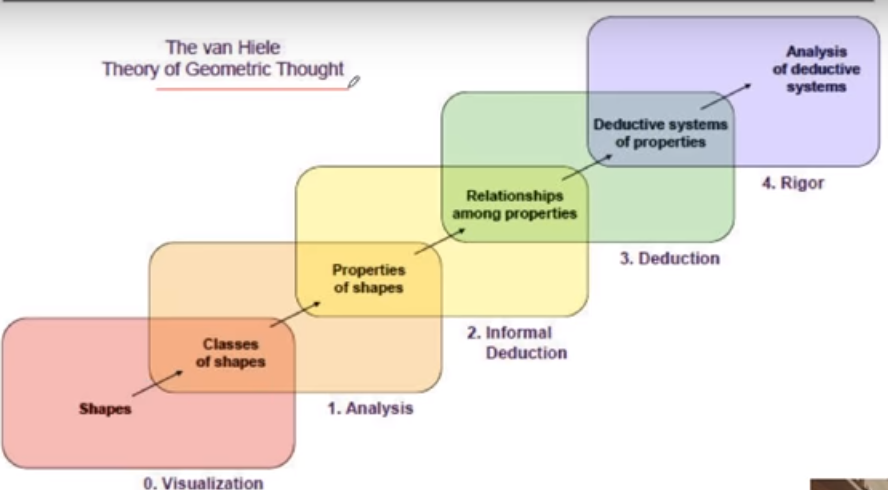
  3. According to van Hiele’s level of geometric thought, the five levels of geometric understanding are visualization, analysis, informal deduction, formal deduction, and rigor.
  4. Students of class7 are asked to classify the quadrilaterals according to their properties. These students are at van hiele level of geometrical thought. – Analysis
  5. Jean piaget highlights the role of proportional reasoning in understanding the concept related to ratio and proportion
  1. A suitable approach to introduce coordinate geometry in class is through the use of
    1. Demonstration using technology integration.

Algebra:

  1. A student cannot able to solve those word problems which involve transposition in algebra. The best remedial strategy is
    1. explain concept of equality using an alternate method.
  2. As per NCF,2005 Mathematics curriculum is ambitious, coherent and important to teachers.
    1. Here ambition refers to seek higher aims of teaching mathematics in school and coherent – teach a variety of mathematics like arithmetic, algebra geometry and data handling.
  3. The following mathematical process is an important aspect of algebra in class 6 – visualization, transposition, and generalization.

Measurements:

  1. A teacher has taught measurement of the area to class 8 children but many of her students are confused between the usage of different units of area and volume. The reason for such confusion in children’s
    1. different units has been introduced all together without relating them with their daily life.
  2. A student was asked to calculate the surface area of a cube. He calculated the volume. The reason for the error in calculation is
    1. the student is not able to understand the concept of surface area and volume.
  3. The correct statement which reflects contemporary view of students errors in math
    1. They are a part of learning
    2. They are a rich source of information
    3. They can guide the teacher in planning her classes.
  4. A teacher of class 7 finds that despite her regular teaching, a student makes errors. The teacher should
    1. study the errors and their possible causes and design her teaching strategy.
  5. errors plays a crucial role in learning of mathematics this statement is true , because errors
    1. provide feedback about the marks they obtained
  6. Class 6 students were given a layout of a house. The students were asked to find out the – 
    1. Perimeter and area of each room
    2. Total perimeter and a total area of the house
      1. This above activity can be used by the teacher as formative assessment task because – the student’s response will help the teacher to diagnose their understanding regarding finding dimensions, calculations, knowledge of formulae for perimeter and area, etc

Skills:

  1. Contemporary understanding of maths pedagogy encourages teachers to do the following, 
    1. develop the skill of systematic reasoning in students
    2. encourage the ability to approximate solutions
    3. create opportunities for students to guess and verify the solutions to problems.
  2. children coming to the school from rural areas have poor
  3. The most suitable strategy to teach the skill of addition of money – Roleplay.
  4. While doing projects in mathematics helps
    1. promoting inquiry skills
    2. enhancing problem-solving skills and
    3. establishing inter-disciplinary linkages.
  5. Neeta a class 7 maths teacher assigns a lot of survey-based projects to mathematics classrooms. The purpose of such activity is 
    1. to promote problem-solving skills
    2. to give an opportunity to students to collect authentic data
    3. to use it as an alternate assessment
  6. Place of mathematical education in the curricular framework is positioned on     2 concerns  –
    1. how it can make them employable after school
  7. As per NCF, 2005 
    1. The narrow aim of teaching mathematics at school is to develop-numeracy related skill and the higher aim is to develop problem-solving skills.
    2. As per 2005, NCF, the goal of mathematics teaching in the school curriculum is that “children learn” “important mathematics”. It implies understanding the appropriate use of learned mathematical techniques.
    3. The mathematics curriculum is ambitious, coherent and teaches important mathematics. here ambitious refers to
      1. seek higher aims of teaching mathematics in school
    4. The twin premises to fix the place of mathematics teaching in our school curriculum are
      1. how to engage the mind of every student and how to strengthen the student’s resources.
    8. null
  8. Which cognitive skill of bloom’s taxonomy is emphasized in this below question?
    1. Solve x+1/2x+3 = 3/8- Analysis.

Multiplication:

  1. The statement which is true for integers
    1. subtraction is not commutative
    2. multiplication is associative
    3. 1 is the multiplicative identity
  2. The method used to prove “the sum of 2 even integers is always even” – Direct proof
  3. “every odd natural number is a prime number”. The following methods of proof which can be used to prove/disprove the above statement  – Method of disproof (Counter positive proof)
  1. After teaching the concept of multiplication to her class, a teacher asked her children to multiply 48 by 4
    1. One of her students solved it orally as “to multiply 48 by 4 we first add 48 to 48 which makes 96 and then add another 96 to reach 192. so the answer is 192.
    2. He/she understood multiplication as repeated addition.

Assessment:

  1. The following statements reflect a desirable assessment practice in the context of maths learning – holding conversations and one to one discussions with children can also be helpful in assessing them.
  2. The purpose of assessment
    1. monitoring students growth
    2. Making instructional decision
    3. evaluating the effectiveness of curriculum
  3. Ankur got zero marks in a word problem on the linear equation in an assessment. The teacher knows he can solve it correctly. The teacher ought to remark in his report, Ankur has a problem in comprehending the language of the question, though he can solve the equation.
  4. Formative assessment in mathematics at primary stages includes identification of learning gaps and deficiency in teaching methods.
    1. One of the teaching methods to be assessed formative is
    2. While teaching ‘shapes’ a teacher can plan a trip to historical places as
      1. Shapes are an integral part of every architecture and such trips encourage connections across children 
  1. Assessment strategy to encourage interdisciplinary in mathematics
    1. Projects and Field trips
  1. In an inclusive mathematics classroom strategy for addressing the needs of visually challenged learners – design alternate teaching-learning assessment methods.
  2. The most appropriate in teaching-learning of data interpretation in class-7 – providing survey reports from newspapers.
  3. ‘Maths lab-activities’ can be used for – Formative assessment only
    1. It is a range of formal and informal assessment procedures employed by teachers during the learning process in order to modify teaching and learning activities and assess student improvement.
  4. Class 6 students were given a layout of a house. The students were asked to find out the – 
    1. Perimeter and area of each room
    2. total perimeter and a total area of the house
      1. This above activity can be used by the teacher as a formative assessment task because – the student’s response will help the teacher
      2. to diagnose their understanding regarding finding dimensions, calculations, knowledge of formulae for perimeter and area, etc,
  5. One of the major reasons for students failure in mathematics at school level is that our assessment process
    1. emphasize on testing of procedural knowledge than mathematization of abilities.
  6. In class 7, a teacher taught the ‘properties of all types of quadrilaterals’. In the class test that followed after the unit, the teacher asked the problems on the construction of quadrilateral.
    1. No one in the class was able to perform in the test. The reason maybe there is a gap between instructions given in class and the assessment conducted.

Upper Primary level:

  1. The method which is most suitable for teaching mathematics at the upper primary level is the problem-solving method.
  2. At upper primary stage the skills promoted by mathematics are
    1. Visualize
    2. transposition
    3. Generalization
    4. Estimation
  1. The most essential in learning mathematics at the upper primary level is exploring different ways of solving a problem
  2. The strategy of questioning used in the maths class at the upper primary level.
    1. helps children to express their thoughts or understanding and thinking critically.
  1. To develop critical thinking among students the least likely is to calculate the volume of a right circular cylinder of a given radius and height
    1. But others that helps in critical thinking
      1. Evaluate 72*73 in the 3 different ways and compare the result
      2. Formulate any 2 situations to represent the equation 7x+3 = 24
      3. a student calculated the volume of a right circular cylinder of radius 3.5 cm and height 10 cm as 38.5 cm^3. Where did she go wrong?
  1. CBSE has recommended a mathematics laboratory as a part of the mathematics curriculum at the upper primary and secondary stages.
    1. The main purpose of a math laboratory is to provide opportunities for hands-on learning.
  2. The most essential aspect of mathematics planning in the upper primary class -provide learning opportunities to allow learners to construct concepts.
  3. Learning mathematics at the upper primary level is about gaining an understanding of mathematical concepts and their applications in solving the problem logically.
  4. Data handling at the upper primary stage focus on –
    1. data collection, organization, and interpretation.
  5. The curricular expectations at teaching primary level include
    1. include developing a connection between the logical functioning of daily life and that of mathematical thinking
    2. develop language and symbolic notations with standard algorithms performing number operations
    3. represent part of the whole as a fraction.
  1. A task assigned to the class 8 students is as follows
    1. An open box is to be made out of a metallic sheet of 50 cm *65 cm. The length and breadth of the box are 30 cm and 15 cm respectively. What is the possible height of the box? Also, find the volume of this box
      1. This task refers to- a higher level of cognitive demand as it requires the use of conceptual understanding that underlines the procedure to complete the task.
  2. Anisha and Amit study in class 7. Anisha told Amit that if the marks in the mathematics of each student in the class are increased by 5, the average would go up by 5.
    1. She further says that it is true for all numbers. Amit does not agree and Anisha proves it by taking the case n, instead of 5. Anisha is using inductive logic.
  3. A teacher in class 7 asks her students to draw circles of different radii. She then asks them to calculate the ratio of circumference to diameter. The students find that this ratio is almost the same in the case of each circle. The teacher using an analytical approach.
  4. Neeta a class 7 maths teacher assigns a lot of survey-based projects to mathematics classrooms. The purpose of such activity is 
    1. to promote problem-solving skills
    2. to give an opportunity to students to collect authentic data
    3. to use it as an alternate assessment
  5. In a textbook of class 7th ” A map is given with a scale of 2cm – 1000km. The actual distance between 2 places in km, if the distance on the map is 2.5 cm the problem is inter-disciplinary nature.
  6. a class 7 teacher  wants to discuss the  following problem in the class:
    1. ” A square is divided into four congruent rectangles. The perimeter of each rectangle is 40 units. What is the perimeter of given square?
      1. Key mathematical concepts required to solve the problem – Meaning of the terms square, rectangle, congruent and perimeter.
  7. A child of class 7 defined the rectangle as follows:
    1. “Rectangle is a quadrilateral whose opposite sides are parallel and equal” The definition reflects that the child
    2. Know some properties, but missed some important to complete the information.
  8. In class 7, a teacher taught the ‘properties of all types of quadrilaterals’. In the class test that followed after the unit, the teacher asked the problems on the construction of quadrilateral. No one in the class was able to perform in the test. The reason maybe there is a gap between instructions given in class and the assessment conducted.
  9. The following mathematical process is an important aspect of algebra in class 6 – visualization.
  10. In class 6, in the unit of understanding quadrilaterals, important results relate to the angle sum property of quadrilaterals are introduced using paper folding activity followed by exercise base on these properties.
    1. At this level proof of the angle property is not given as the student of class 6 is at Van-Hiele of level-1 analysis.
  11. In class 6 teacher gave a topic for debate as a formative assessment. Hindu Arabic numerals are more powerful than roman numerals objective of this task is to assess analysis.
  12. From the textbook of class 6
    1. “Express the following statement through linear expression: Neha has 7 more toffees than Megha. If Megha has x toffees, how many toffees does Neha have?
    2. Which competence of bloom’s cognitive domain is referred to the above equation? – comprehension.

Math Communication:

  1. children coming to the school from rural areas in the context of mathematics
    1. they have poor communication skills in mathematics.
  2. The most appropriate aim of encouraging mathematical communication in the classroom is
    1. Children should be able to use a precise language while talking about mathematical statements and using them.
  3. Using technology based games involving factorisation of numbers or formation of new shapes by joining 2D shapes etc, 
    1. enhances the students ability to understand the concepts better as as they are able to explore, observe and infer at their own pace.
  1. Place of mathematical education in the curricular framework is positioned on     2 concerns  –
    1. What mathematics education can do to improve the communication skills of every child and how it can make them employable after school.
  2. Communication in mathematical class refers to developing the ability of 
    1. organize, consolidate and express mathematical thinking.
  3. A teacher conducted a debate in the class on the following topic:
    1. “Zero is the most significant number”. She encouraged every child to express his/her view on the topic. The teacher is making her classroom more communicative and reflective.

Math Test:

  1. The purpose of the diagnostic test in mathematics is to know the gaps in children’s understanding.
  2. One of the major reasons for students failure in mathematics at school level is that our assessment process needs to
    1. emphasize on testing of procedural knowledge than mathematization of abilities.
    2. So we need to gove more weightage to formative assessment than summative assessment to recognize the individual difference of learners.
  3. In class 7, a teacher taught the ‘properties of all types of quadrilaterals’. In the class test that followed after the unit, the teacher asked the problems on the construction of quadrilateral. No one in the class was able to perform in the test. The reason maybe there is a gap between instructions given in class and the assessment conducted.
  4. Some students of your class are repeatedly not able to do well in mathematics exams and tests. As a teacher, you would-Diagnose the cause and take steps for remediation.
  5. Remedial teaching is helpful for removing the learning difficulties of weak students.

Jean Piaget:

  1. According to Piaget when the child is at the formal operational stage it is appropriate to introduce ratio and proportions
  2. A child of class 6 is able to find the solution of given linear equations but is not able to solve word problems. The child is at Piaget’s pre-operational stage
  3. According to Jean Piaget’s theory,
    1. introduction of a linear equations and learning techniques of solving it are assimilation, and extending the concept to a pair of linear equations may raise the problem of accommodation.
  4. Algebra is introduced in the middle classes. According to Piaget’s theory of cognitive development, it is appropriate to introduce algebra at this stage as
    1. the child is at the concrete operational stage and he can understand conceptualize concrete experience by creating a logical structure.

Problem Solving :

Divisibility:

  1. If the 7 digit number is 134x58y is divisible y 72 then the value of (2x+y) is
    1. Divisible by 72 is divisible by 2 and 3
    2. so y must be 0,2,4,6,8,
    3. If divisible by 3, 
      1. 1+3+4+x+5+8+y must be divisible by 3
      2. 21+x+y must be divisible by 
        1. For y=0, x=0,3 then 2x+y = 0,6
        2. For y=2, x=1 then 2x+2 = 4
        3. For y=4, x=2 then 2x+4 = 8
  2. If an 8 digit number 30x0867y is divisible by 88, then what is the value of (3x+y)
    1. Divisibility by 88 is divisible by 8 and 11
      1. Since divisible by 8, 670/8 = has remainder but 672/8 has no remainder, so y = 2
      2. (18+x) – 10 =11 therefore x = 3
    2. Sub x and Y, 3(3)+2 = 11.

Age:

  1. Abhi is twice as old as as his daughter, Five years ago, his age was 4 times his daughter’s age. If the present age off the daughter is x years then:
    1. Let the present age of abhi be Y years and age of his daughter be X years
    2. By Qtn,
      1. y = 2x
      2. Now , 5 yrs ago
      3. y-5 = 4(x-5)
      4. 2x-5 = 4(x-5)
  2. Aman is now fifteen years younger than abdul. After 6 yars, abdul will be twice as old as a man. will be then. If the present age of abdul is x years, then we have
    1. Condition: 2(x+6) = (x-15)+6 = 2(x+6) = (x-9)
NowAfter 6 years
Amanx-15(x-15)+6
Abdulxx+6
  1. Rani, who is y years old at present is x years older than Hamid. 15 years ago, hamid’s age was 1/4 of the age of Rani. True statements are
    1. Present age of rani = y year,
    2. 15 yrs before rani’s age = y-15
    3. Hamid’s age = y-x
    4. 15 yrs before Hamid’s age = y-x-15, According to question
    5. y-x-15 = 1/4(y-15), 3y-4x = 45.

Area:

  1. If each edge of a solid cube is increased by 150 %, the percentage increase in the surface area is
    1. Surface area of cube = 6 x L x L
    2. If the edge is increased by 150% then the new edge = 1L + 1.5 L = 2.5L
    3. Therefore, the increased surface area = 6 x 2.5L x 2.5L = 37.5
    4. Therefore, the % increase would be     {(37.5 – 6)/6} x 100 ={ 31.5/6}x 100  =  5.25 x 100 = 525
  2. The base of an isoceles triangle ABC is 48 cm and its area is 168 cm^2. The length of one of its equal sides is
    1. 168 = 1/4*a root /-4b^2 – a^2, root/- 4b^2 – (48)^2 = 168*4/48
    2. 14 = root/-4^2-(48)^2
    3. Squaring both sides,
      1. 196 = 4b^2 – (48)^2, b^2 = 196/4 +48*48/4, b^2 = 49+12*48
    4. b^2 = 49+576 = 625, b = root/-625 = 25 cm
  3. The area of a square is 16/pi of the area of a circle. The ratio of the side of the square to the diameter of the circle is
    1. Area of circle = pi*r^2, Diameter of circle = 2*r,
    2. Area of square – 16/pi *pir^2
    3. a = root /-16r^2 side of square = 4r
    4. Side of the square/diameter of the circle = 4r/2r = 2/1 = 2:1
  4. 4 times the area of the curved surface of a cylinder is equal to 6 times the sum of the areas of its bases. If its height is 12 cm, then its volume in cm^3 is
    1. Area of curved surface = 2*pi*r*h,
    2. area of base of cylinder = pi*r^2
    3. By question,
      1. 4*2pirh = 6*pi*r^2, 4*2pi*r*12 = 6*pi*r^2
      2. r=8 cm.
    4. Volume of cylinder = pi*r^2*h = 768 pi
  5. The perimeter of a trapezium is 58 cm and sum of its non-parallel sides is 20 cm. If its area is 152 cm^2, then the distance between thee parallel sides in cms is
    1. Sum of parallel sides (a+b)= 58 -20 = 38 cm
    2. Area of trapezium = 1/2 *sum of parallel lines(a1+a2)*h , 1/2*38*h = 152, 19h = 152 and h=8 cm.
  6. The ratio of the areas of 2 equilateral triangle is 16:9. If the perimeter of the smaller triangle is 63 cm, then how much larger is a side of the larger triangle than a side of the smaller triangle
    1. root/- 3/4a1^2/ root/- 3/4 a2^2 = 16/9 
    2. a1/a2 = root/- 16/9 = 4/3
    3. Given, 3 a2 = 63 cm, so a2 = 21 cm ||ly a1 =28 cm
    4. So, larger /\ – smaller /\ = 28 -21 = 7 cm.
  7. The area of a triangle is equal to the area of a circle whose perimeter is 6 pi cm. If the base of the triangle is 8 cm, then its corresponding height is
    1. 1/2 bh = pi*r^2, given 2 pi r = 6*pi so, r= 3 cm
    2. 1/2 *8*h = pi*3^2 so, h= 9pi/4  = 2.25 pi
  8. ABCD is a quadrilateral in which D =40 cm. The lengths of thee perpendiculars drawn from the opposite vertices on D are 16 cm and 12 cm. The area of the quadrilateral is
    1. Area of quad = 1/2*d(h1+h2) = 1/2*40(16+12) = 560 cm ^2
  9. A square and a circle are formed using pieces of wire of length 5024 cm each. The ratio of the area of the square to that of the circle is
    1. 4 a = 5024, a =1256 so, 2*pi*r = 5024,
    2. r = 2512/pi
    3. a^2:pi*r^2 = 1256*1256 : 2512 *2512 / pi
    4. pi : 4
  10. On increasing each side of a square by 25% the increase in area will be
    1. |_| sqre 100 —-> 125 (4—>5) – Since 25% increase
    2. therefore % increase in area = 25-16/16 *100 = 9/16*100 = 225/4 = 56.25%
  11. There are 2  pieces of wire each of length 30 cm. Using one piece a square is formed and from the other piece a triangle of sides 5 cm, 12 cm and 13 cm is formed. The ratio of the area of the triangle to that of the square is
    1. For |_| re 4a=30 a=15/2 and
    2. For /\ gle 1/2 b*h = 1/2*12*5 = 30 cm
    3. Area of /\gle : area of |_| qre 30:(15/2)^2 = 8:15
  12. To fill a rectangular tank of area 700 m^2, 140 m^3 of water is required. What will be the ht of the water level in the tank?
    1. Vol of tank – l*b*h =140,
    2. given l*b = 700
    3. h = 140/700 = 1/5 mts since 1 mts = 100 cm, 1/5 *100 = 20 cm.
  13. The area of trapezium is 105 cm^2 and its height is 7 cm. If one of the parallel sides is longer than the other by 6 cm then the length of the longer side in cm is
    1. Area =1/2 *h*(a1+a1)
    2. 105 = 1/2*7*(x+x+6)
    3. 210 = 7(2x+6)
    4. x=12
      1. length of longer side = x+6 = 12+6=18 cm
  14. The curved surface area of a right circular cylinder of base radius 3 cm is 94.2 cm^2. The volume of the cylinder is 
    1. CSA of cylinder = 2pi r *h
    2. h = 94.2 / 2*3.14*3 = 5 cm
    3. Vol of cylinder V = pi*r^2*h = 141.3 cm^2
  15. The area of a quadrilateral is 227.2 cm^2 and the length of the _|_ lars from the opposite vertices to a diagonal are 7.2 cm and 8.8 cm. What is the length of the diagonal? – 28.4 cm
    1. 1/2*d(h1+2)

Triangle:

  1. If the angles in degrees of a triangle are x,3x,+20, and 6x the triangle must be
    1. x+3x+20+6x = 180
    2. 10x+20 = 180 so x= 16*, Angle = 6x = 6*16 = 96*
    3. Hence, triangle is obtuse.
  2. In triangles ABC and DEF |_C = |_F AC=DF, and BC =EF. If AB= 2x-1 and DE = 5x-4 then the value of x is
    1. Since congruent triangles ABC and DEF
    2. 2x-1 = 5x-4
    3. so x = 1
  3. One side of a triangle is 5 cm and the other side is 10 cm and its perimeter P cm, where P is an integer The least and the greatest possible values of P are respectively
    1. Sum of two side > third side
    2. 5+10 > x  = x<15
    3. Also, 5+x>10, 
    4. so x > 10-5 where x>5
    5. P = 10+5+x
      1. For maximum, P<5+10+15 = P<30
      2. For minimum, P>5+10+5 = P>20
      3. Hence,  21<_P<_29
  4. The sides of 4 triangles are given below: Which of them forms a right triangle?
    1. 11 cm, 60 cm, 61 cm
  5. Not a pythogorean triplet?
    1. 8,15,17   11,60,63   13,84,85   7,24,25
      1. C^2 = a^2 + b^2
      2. 17^2= 8 ^2 + 15^2 , so 8,15,17 are pythogorean triplet
  1. Two sides of a right triangle measure 15 cm and 17 cm. True statements about the length of the third side of the triangle
    1. It is less than 10 cm
    2. It is between 20 cm and 23 cm
      1. Properties of a triangle: 1. 2 sides sum greater than the third side and 2 sides difference cannot be lesser than the 3 rd side 17-15<x<17+15.
    1. case-1:
      1. If x is the largest side, apply pythogoraus theorem,
      2. x = root of 17^2+15^2 = 22.67
    1. Case- 2
      1. If x is not the largest side then 17 is hypotenuse
      1. x^2 = 17^2-15^2 = 64, so x= 8 cm
  2. If one angle of a triangle is 110 deg, then the angle betweeen the bisectors of other two angle measure
    1. Solution:
      1. |_A+|_B+|_C = 180, |A+|C = 180-110 =70 so |_A/2 + |_c/2 = 35*
      2. So, |_AOC = 180 -35 = 145*
        1. Let X and Y be the bisected angles, so in the original triangles , sum = 180*
        2. 110*+2x+2y = 180*
        3. x+y = 35*
        4. In the smaller triangle consisting of original side opposite 110*
        5. Bisected angle = 180 – 35 = 145*
  3. In triangle ABC, AB= 4 cm, AC=5 cm, and BC= 6cm. In triangle PQR, PR=4 cm, PQ=5 cm and RQ= 6 cm. triangle ABC is congruent to /\ le ABC is congruent to /\ PRQ.
  4. The ratio of the areas of 2 equilateral triangle is 16:9. If the perimeter of the smaller triangle is 63 cm, then how much larger is a side of the larger triangle than a side of the smaller triangle
    1. Soln: root/- 3/4a1^2/ rooot/-3/4 a2^2 = 16/9 
      1. a1/a2 = root/- 16/9 = 4/3
      2. Given 3 a2 = 63 so a2 = 21 ||ly a1 =28 cm
      3. So, larger /\ – smaller /\ = 28 -21 = 7 cm
  5. In a triangle AC, D is the mod point of BC and angle C = 40 deg.A is joined to D and AD = BD. The measurement of angle BAC is 
    1. Since AD=BD complementary angles in angle ABD are equal
    2. Ang D= ang A + ang C = 40 + 40 =80 so In triangle ABD( (50+50+80) ang A = 50 and Ang B= 50
    3. therefore |_Ang BAC = 90*
  6. The perimeter of a /\gle is 12 cm. If all the 3 sides have lengths(in cm) in integers then how many such different /\gles are possible. 2

Quadrilateral:

  1. In a quadrilateral ABCD, < D = = 60 and <C= 100. The bisectors of angle A and D meet at point P. the measure of angle < APB is
    1. Solution:
    2. Angle A is opposite to Angle C. Since angle C is 100 then angle A would be 80
    1. Bisector of angle A and D meets at P. Then the points A,P and D forms a triangle. Thus the angle
    2. angle  APB would be equal to
    1. <APB = 180 – 60 – 40 = 80*
  2. The angles of a quadrilateral are in the ratio 2:3:5:8. The sum of the supplement of the largest angle and the complement of the smallest angle is
    1. 2x+3x+5x+8x =360*, x= 360/18 =20
    2. 2:3:5:8 –  smallest complement – 90-(2*20) = 50
    3. Largest supplement – 180- (8*20) = 20
    4. Sum = 50+20 = 70*
  3. The measure of an angle for which the measure of the supplement is 4 times the measure of complement is
    1. let angle is theta, then its complement (90-teta)
    2. theta + 4(90-theta) = 180
    3. 360 – 3 theta = 180 theta = 60*
  4. The angles of a quadrilateral are in the ratio 3:5:7:9. What is the difference between the least and the greatest angles of quadrilateral?
    1. 24x = 360
    2. x=15 deg, So diff = 135-45 = 90*
  5. In which of the following cases, the construction of a quadrilateral ABCD is not possible
    1. BC = 4.5 cm, AD = 5.5 cm, CD = 5 cm, AC = 5.5 cm and BD = 7 cm
    2. AB=3.5 cm, angle =125 deg, ang C=80 deg, BC= 5.5 cm and CD = 5 cm
    3. AB = 4 cm, BC = 6 cm, AC = 8 cm, AD = 5.5 cm and DC = 5 cm
    4. AB = 6 cm, C = 9.5 cm, ang A = 75 deg, ang B = 150 deg and ang C = 140
  6. The construction of a quadrilateral ABCD is not possible
    1. AB=5 cm, BC= 3.5cm, CD = 5 cm, AD= 3cm and AC =8.5 cm
    2. AB = 5 cm, BC = 4 cm,|_A = 60* |_B = 105* and |_C = 105*
    3. AB=BC=3 cm, AD= 5 cm, |_A = 90* and |_B = 105*
    4. AB = 4.5 cm, BC = 4 cm, CD = 6.5 cm, AD = 3 cm, and BD = 6.5 cm.

Cube and cuboid:

  1. 42 cubes each of side 1 cm are glued together to form a solid cuboid. If the perimeter of the base of the cuboid is 18 cm then its height in cm is
    1. peri = 18, Area of base = 1/2*42, ht = 21-18 = 3 cm
  1. The internal base of a rectangular box is 15 cm long and 12 1/2 cm wide and its height is 7 1/2 cm. The box is filled with cubes each of side 2 1/2 cm. The number of cubes will be
    1. Vol of cuboid = 15*12 1/2 *7 1/2 (l*b*h) = 1406.25
    1. vol of cub = a^3 = 2 1/2 *2 1/2 * 2 1/2  = 15.625
    2. Number of cubes = 15*25/2*15/2 / 5/2*5/2*5/2 – 15*25*15*2 / 5*5*5 = 90 cm
  2. A tank is in the form of a cuboid. It holds a maximum of 540 m^3 water. If the tank is 8 m long and 15 m wide, then how many mts deep must the water be when the tank is 2/3 full?
    1. V= l*b*h
    2. h = 9/ 2 m
    3. Depth = 2/3 of 9/2 = 3 mts
      1. Method 2
      2. 540 * 2/3 = 8*15*h = 3 mts
  3. The total surface are of a cuboid is 194 m^2. If its length is 8m and breadth is 6 m. then what is its volume(in m^2)?
    1. Let ht be h
    2. TSA = 2(lb +bh+lh)
    3. 194 = 2(8*6 + 6h +8h)
    4. h = 49/14 = 7/2
    1. V = l*b*h = 8*6*7/2 = 168 m^2
  4. A godown is in the shape of a cuboid whose length, breadth and ht are 56 mts, 42 mts, and 10 mtss. How many(maximum) cuboidal boxes each measuring 2.8m*2.5m*70 cm
    1. 4800
      1. 56*42*10 / 2.8*2.5*0.7 = 4800.

Cylinder:

  1. The diameter of a cylindrical jar is increased by 25%. By what % must the height of the jar be decreased so that there is no change in its volume?
    1. If diameter increased radius also increased
    2. r1 = 100r and h1 = 100h
    1. V1 = 1000000 pi r^2h
    2. new v2 = (125r)^2 pi.h2 = 
    3. h2 = 1000000h/15625 = 64h = 100h-36h
  2. Circumference of the base of a right circular cylinder is 44 cm and its height is 15 cm. The vol of the cylinder is
    1. 2*pi*r = 44, r=44*7/ 2*22 = 7 cm
    2. pi*r^2*h = 22/7*7^2*15 = 770 cm^2
  1. Circumference of the base of a right circular cylinder is 44 cm and its ht = 15 cm. Find the vol of the cylinder
    1. 2 pi r= 44
    2. r = 44/2 pi = 7
    3. Vol = pir^2h = 22/7*7^2*15 = 2310.
  1. On recast the radius of an iron rod is made 1/4th. If its volume remains constant, then the new lngth will become – 
    1. V= pir^2l
    2. V1 = pi (r/4)^2 l1
    3. l=l1/16 so new length of the cylinderical rod formed after recasting is 16 times the original length.
  1. The radii of the bases of two cylinders are in the ratio of 2:3 and their heights are in the ratio of 5:3 . The ratio of their volumes is
    1. Let the radii be 2x and 3x and the heights would be 5h and 3h.
    1. Then the ratio of volumes would be    (2x) 2 (5h) / (3x) 2 (3h) = 4 x 5 / 9 x 3 = 20/27
  2. 2 sheets of paper with a measure of 22 cm y 28cm are taken. Each sheet is then rolled into a cylinder, one having the height 22 cm and the other height 28 cm. The difference in their volumes will be
    1. If 2*pi*r = 28, h=22 cm so vol = pi*r^2*h
    2. 294
  3. A 22 cm*8 Cm rectangular piece of paper is folded and taped without overlapping to make a cylinder of ht 8 cm. The volume of the cylinder so formed is
    1. 2 pi r = 22 cm, If ht = 8 cm, r = 7/2
    2. Vol = pi*r^2*h = 22/7*7/2*7/2*8 = 28*11 = 308 cm^3
  4. The circumference of the bsae of a right circular cylinder is 528 cm and  its ht is 2 m. What is the vol of the cylinder? – 4.4352 m^3

Polygon:

  1. The sum of all interior angles of a regular convex polygon is 1080. The measure of each of its interiror angles is 135.
    1. The sum of all angles of a regular convex polygon = (n-2)180
    2.  (where n is the number of sides of a regular hexagon)
    3. 1080 = (n-2)180
    4. (n-2) = 1080/180
    5. (n-2) = 6
    6. n=  8
    7. Hence, the polygon is regular octagon and the measure of each angle is 1080/8 = 135
  2. The sum of all interior angles of a polygon is 1440 degree. The number of sides of the polygon is
    1. Interior angle of a polygon = 180(n-2), By question = 180(n-2) = 1440
    2. 180n -360 = 1440, 180n = 1440+360 
    3. 180n = 1800,n = 1800/18 = 10
  3. The measure of each interior angle of a regular convex polygon is 156 deg. The number of sides of the polygon is
    1. Exterior angle = 180-156 = 24*
    2. Number of sides = 360*/ exterior angle = 360/24 = 15
  4. If the number of sides of a regular polygon is ‘n’ then the number of lines of symmetry is equal to
    1. n/2,n^2, 2n, n
  5. How many sides does a regular polygon have if its each angle is of measure 108 deg
    1. Sum of all interior angle of regular polygon = (n-2)*180
    2. But for measure of each angle = (n-2)*180/n = 108
    3. 5n-10=3n so n=5
  6. A regular polygon has 10 sides has 10 lines of symmetry

Reference:

  1. https://books.google.co.in/books?id=JjauDwAAQBAJ&lpg=RA3-PA21&ots=xvtYOXF2YD&dq=CTET%20feb%202014%20Biosphere%20reserves%20are%20the%20areas%20which%20helps%20to%20maintain&pg=RA2-PA31#v=onepage&q=CTET%20feb%202014%20Biosphere%20reserves%20are%20the%20areas%20which%20helps%20to%20maintain&f=false
  2. https://books.google.co.in/books?id=g2FjDwAAQBAJ&lpg=PA71&ots=8UUnuH9Izy&dq=CTET%20sep%202014%20Normal%20temperature%20of%20human%20body%20on%20the%20celsius%20scale%20and%20fahrenheit%20scale%20is&pg=PA71#v=onepage&q=CTET%20sep%202014%20Normal%20temperature%20of%20human%20body%20on%20the%20celsius%20scale%20and%20fahrenheit%20scale%20is&f=false – Refer from page 12
  3. https://books.google.co.in/books?id=fWFjDwAAQBAJ&lpg=PP126&dq=The%20%20project%20%20method%20of%20teaching%20%20is%20%20best%20%20associated%20with%20the%20philosophy%20of&pg=PP133#v=onepage&q&f=true
  4. https://www.google.co.in/books/edition/CTET_Previous_Year_Solved_Papers_for_Mat/E4LADwAAQBAJ?hl=en&gbpv=1 – CTET 2016 solved papers